Wietse M. Boon

Mathematician

Published version (open access)

Key ideas

  • We rewrite the Stokes equations as a vector Laplacian and reformulate the equations in terms of vorticity, velocity, and pressure.
  • The equations are discretized using low-order finite element spaces that conform to the de Rham complex.
  • Using a low-order quadrature rule, the vorticity is eliminated and we are left with the Raviart-Thomas finite element pair of lowest order.
The three finite elements used for the vorticity, velocity, and pressure in 3D. The vorticity space (yellow) is eliminated through a structure-preserving quadrature rule.

Main findings

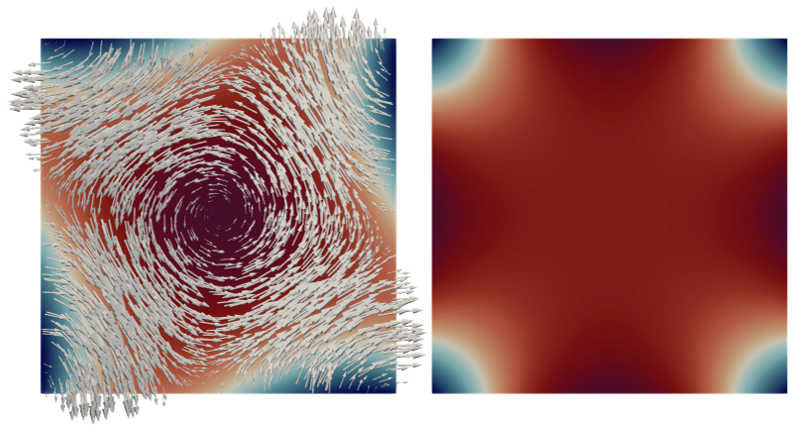
  • First order (optimal) convergence is shown in $H(curl) \times H(div) \times L^2$.
  • The pressure is invariant to the use of the quadrature rule.
  • In 2D, the vorticity variable is invariant and converges quadratically
  • The flow field is pointwise solenoidal and the method is pressure robust.
Stokes flow calculated using the multipoint vorticity method with a rotational forcing term. The velocity is superimposed on the vorticity on the left and the right shows the pressure.